Q*Bert Topology for Isometric Maps

I mentioned this somewhere else, so I figured I'd explain.  Certain things make maps easier to draw, others make them much more time consuming.

One the best ways to slow down mapping is doing research to make sure the map is accurate relative to some pre-existing source material.  It's no accident that most of us armchair cartographers draw a map of our dungeons and then stock them - that's way easier than writing a description of a large dungeon and then trying to produce an accurate map of it.

But tied for second place in terms of taking a lot of time is fiddling around with a 3D orientation, trying to make a complex, overlapping object comprehensible.

(An astute patron pointed out that I missed map #3 in my series - Tannoch the fifth map, but it's numbered 06.  That's because map #3 fell victim to both of these problems at the same time and I couldn't get the map done. When I level up I'll tackle it again.)

Q*Bert Topology

What makes 3D maps hard is when you need to show something that's behind something else.  If you're drawing the map first, why not simply avoid this entirely?  A topology where nothing overlaps is one where everything is equidistant from the viewer, which, in an isometric map, corresponds roughly to looking down at a staircase at a 45-degree angle.  Q*Bert!

(For the young ones in the crowd, this isn't a sticker, but was once state-of-the-art arcade fun.)  As you can see, in the Q*Bert topology, nothing overlaps, so you can get a lovely sense of the map being 3D, with lots of interesting elevation changes, without the inconvenience of trying to render overlapping.

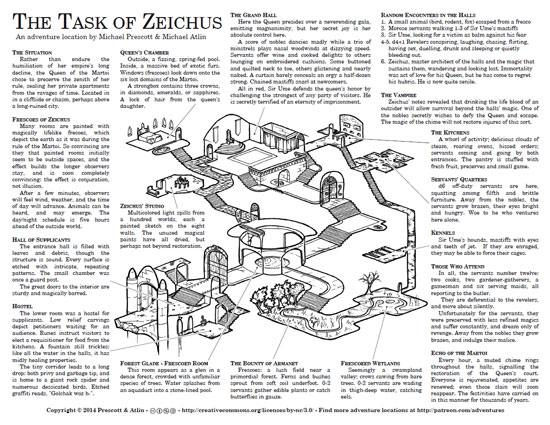
Here's my best example of this, the map from the next adventure, "The Coming of Sorg":

As you can see, this follows the Q*Bert topology very closely:

If all the maps looked like this, it would of course get very dull.  But it's a useful topology to keep in your back pocket for those Sunday afternoon noodling-around-with-graph-paper sessions!

The Task of Zeichus

A fragment of song lyrics got me thinking about barely restrained hostility, a veneer of respectability.  That, plus some super awesome isometric graph paper produced this next adventure.

Here's the link to the high-res art!  Like the adventure, it's cc-by-nc.

'Task' can be plopped pretty much anywhere in a campaign world; it's the sole surviving remnant of something much older, a baroque and decadent time capsule. When I say it might be near some ancient ruins, I mean rocks that you can barely tell were once structures. The domains of the Martoi, whatever they were, are long gone.

The halls are not obviously hostile to visitors, at least not at first.  The revelers will probably be fascinated by novelty, if the party doesn't prove to be crude vandals, and the Queen has a tradition of hospitality toward supplicants while they wait in hopes of an audience - that extends at least as far as the hostel.

The revelers aren't pushovers, however! Although fallen into decadence, they're the elite of a violent society and are familiar with brutality of every sort.  They're also well aware of the chime. If it does come to blows, they'll be more than willing to suffer an apparent setback to deliver a surprise attack an hour later!  But once the dust settles, they will be more than willing to write off fighting as a diverting pastime, as long as honor is satisfied.

The real danger is, of course, the vampire.

Don't forget to check out the others in the series!